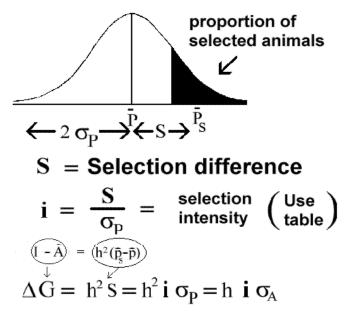
Figure 8.1. Shows a graphic interpretation of the selection difference
(S), the
selection intensity (i) and selection response (delta G) by use of individual
selection.
 |
Figure 8.1 shows a normal distribution of a quantitative trait. A limit of
the selection can be defined according to the proportion being used for breeding.
S designates the difference between the population average and the mean value of the selected animals. If a specific group has been selected,
its actual mean
value is calculated and then S can be calculated. When a certain proportion of animals
is used in breeding, the standardized difference in the selection can
be seen in a table when using truncation selection.
This difference is called
selection intensity, as shown
in Figure 8.1, it can be estimated as S/sigmaP, sigmaP
being the phenotypic standard deviation.
Figure 8.1 also shows the connection between estimated breeding value and the simple formula for selection response R = h2 * S, given in section 6.4, and its relation to the estimation of breeding value by means of its own phenotype, cf. section 7.2.
Below is a table of 'i' calculated on the basis of the proportion of animals selected for breeding.
Proportion Intensity Proportion Intensity Proportion Intensity of animals i of animals i of animals i for breeding for breeding for breeding ----------------------- ----------------------- ----------------------- 1.00 0 0.90 0,20 0.09 1,80 0.008 2,74 0.80 0,35 0.08 1,85 0.006 2,83 0.70 0,50 0.07 1,91 0.004 2,96 0.60 0,64 0.06 1,98 0.002 3,17 0.50 0,80 0.05 2,06 0.001 3,38 0.40 0,97 0.04 2,15 0.0008 3,43 0.30 1,14 0.03 2,27 0.0006 3,51 0.20 1,40 0.02 2,42 0.0004 3,61 0.10 1,76 0.01 2,67 0.0002 3,79
An example of the use of the table: If 30% of the animal is used for breeding, i is 1.14.